Cumulative Gasket Pictures

Exercise Solutions

The vertices of the equilateral gasket are

V1 = (0,0), V2 = (1,0), and V3 = (1/2,sqrt(3)/2) approx (0.5,0.866).

Recall the midpoint formula: the midpoint of (a, b) and (c, d) is ((a+c)/2, (b+d)/2).

Moving half-way between (x, y) and V1 is just taking the midpoint of (x, y) and V1. That is,

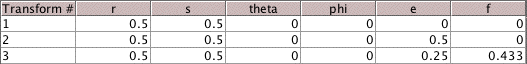
(x, y) -> ((x+0)/2, (y+0)/2) = (x/2, y/2).

Moving half-way between (x, y) and V2 is just taking the midpoint of (x, y) and V2. That is,

(x, y) -> ((x+1)/2, (y+0)/2) = (x/2, y/2) + (1/2, 0).

Moving half-way between (x, y) and V3 is just taking the midpoint of (x, y) and V3. That is,

(x, y) -> ((x+(1/2))/2, (y+(sqrt(3)/2))/2) = (x/2, y/2) + (1/4, sqrt(3)/4).

That is, the chaos game rules for the vertices of the equilateral traingle are equivalent to the IFS ruls for the equilateral gasket.

Return to Exercises.