Nonlinear Tessellations

Background

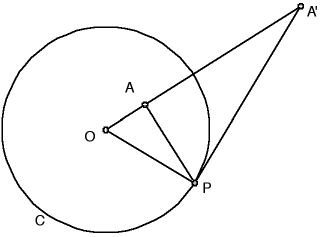
Constructing the Inverse of a Point

Construction Suppose the point A lies within the circle C.

Draw a line through center O and the point A.

Draw a line perpendicular to OA through point A.

Let P denote the intersection of this perpendicular and the circle.

Draw the tangent to C at P and label A' the intersection of this tangent with OA.

This point A' is the inverse of the point A with respect to the circle C.

The same diagram, reversing the places of A and A', constructs the inverse of a point A outside the circle C.

This was Appolonius' original construction. We prove this gives the more familiar condition OA*OA' = OP².

Proof Triangles OA'P and OPA are similar.

Consequently, OA/OP = OP/OA'.

Cross-multiplying gives the familiar condition.

Return to Basic constructions.