| Most musical instruments have natural "sounds," even if we are
not familiar with the
particular composition, a tape of violin music played at the wrong speed will not sound
like violin music. The character of the violin music has a natural time scale. On the
other hand, there are kinds of "music" that sound pretty much the same regardless of
the speed at which the tape is played (within limits, of course, if we play the tape
so fast that only dogs can hear it, then the character of the sound has changed).
These are called scaling noises. How can such noise possibly
sound? |
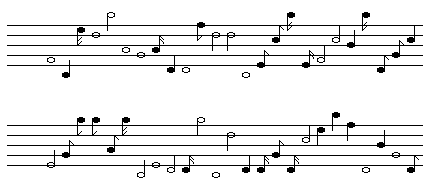
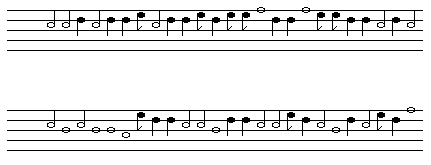
| The simplest example, called
white noise, is easy to generate. Set a range
of note durations (for example, whole, half, quarter, eighth, sixteenth), and a range
of tones. Then use a random number generator to select the duration and tone of each
note in sequence. Except for a uniform change of the duration of the notes, playing
this composition at a different speed will give something sounding about the same.
This kind of composition wanders all over the place and does not sound very interesting.
Indeed, some people find these compositions unpleasant: there is no relation of one
note to the next, no pattern or familiarity one can perceive. Yet this was the
underlying construction of some of John Cage's stochastic music experiments
in the 1960s. |
|
|
| Thanks to Harlan Brothers for the midi files of these tunes. |
| Another example of scaling noise is called
Brownian noise, a name suggested by
Brownian motion, the motion
visible in a drop of water when pollen grains are buffeted
by water molecules. So to generate Brownian noise
use the random number generator to produce not the durations and the tones of the
notes, but rather the changes in the durations and tones. The analogy is that the
water molecules executing thermal (white) motion affect changes in the moton of
the pollen grains. With the obvious considerations for changes of durations, playing
a tape of Brownian noise at a different speed still sounds like Brownian noise,
so this is a scaling noise. While this, too, wanders all over the staff, the
steps are very small, and playing a Brownian composition has the expected effect.
It is too predictable, too correlated, in a word - boring. |
|
|
| To see how the third (and by far the most interesting) kind
of scaling noise differs from the other two, we use the
power spectrum. For white
noise the power is unrelated to the frequency, so we say
this noise has a 1/f0 distribution. (Since f0 is a constant,
this is just another
way of saying the power is independent of the frequency.) On the other hand, Brownian
noise exhibits a 1/f2 power spectrum. This is not obvious, but very roughly it works
like this: to get a very high frequency note we would have to see a lot of successive
increases in pitch, and this is very unlikely (though not impossible) since the
changes in tone are determined randomly, allowing increases as well as desceases.
A third type of scaling noise is called 1/f noise, and its power spectrum has a 1/f
shape. This type of noise occurs in many, many
natural situations.
Playing 1/f noise produces some interesting results: it is not
so random as white noise, and not so predictable as Brownian noise, but has some
elements of both. |
|
|
| We present two examples of this type of analysis of music.
One by Voss and Clarke, the other by
Andrew
and Kenneth Hsu. So we see there are still more ways in which music exhibits fractal
properties - here through the presence of power laws. |