Dimension by Box-Counting

Background - Dimensions of the Sierpinski Gasket and Koch Curve

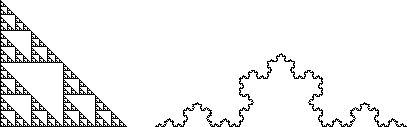
The gasket (left) is made of three copies of itself scaled by 1/2. The Koch Curve (right) consists of four copies of itself each scaled by 1/3.

From the scaling relation N(r) = (1/r)^d we see

for the gasket 3 = (1/(1/2))^d = 2^d and

for the Koch curve 4 = (1/(1/3))^d = 3^d.

For the gasket, taking log of both sides gives log(3) = dlog(2), so d = log(3)/log(2), about 1.58.

For the Koch curve, the corresponding calculation gives d = log(4)/log(3), about 1.26.

We can apply this formula to any self-similar object, but not to natural fractals, physical objects (necessarily not exactly self-similar) that twist and turn so much and on so many scales that we can consider them fractals. To handle these we need a more generally applicable method of computing dimensions.

Return to Background.