Pascal's Triangle and Its Relatives

Background

A Quick Sketch of Some Group Theory

The order in which elements of a cyclic group are added is irrelevant: 1 + 2 = 2 + 1, for example.

However, for symmetry groups that include rotations and reflections, the order of the operations can matter.

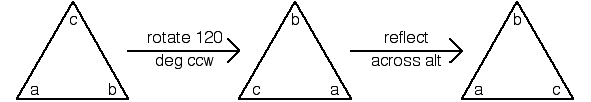
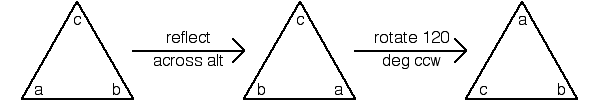
For example, label the vertices of an equilateral triangle, so we can keep track of the positions of the vertices.

Consider the symmetry operations rotate 120 deg about the center and reflect across the altitude.

Certainly, the order of the transformations has an effect on the outcome.

So in general, we must consider groups in which the order of the operations can matter: a + b need not equal b + a.

Return to Some group theory.