Pascal's Triangle and Its Relatives

Exercises

Other mods

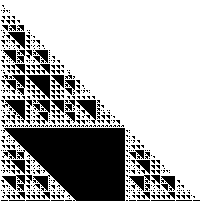
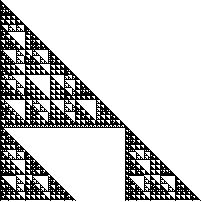
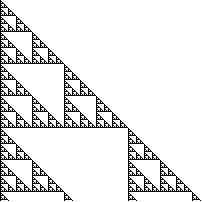
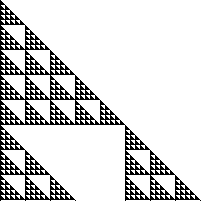
7. (mod 10) Shading the boxes of Pascal's triangle with numbers congruent to

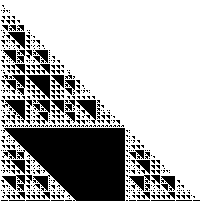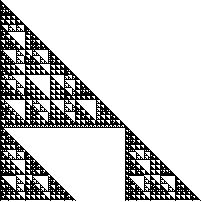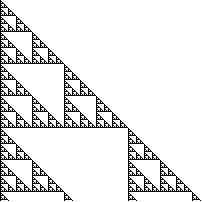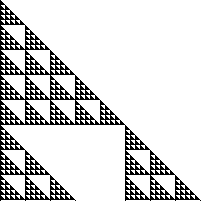0 (mod 10)	1, ... 8, or 9 (mod 10)	1, 2, 3, 4, 6, 7, 8, or 9 (mod 10)	1, 3, 5, 7, or 9 (mod 10)

Observe Z10 has two proper subgroups,

H1 = {0,5}, ispmorphic to Z2, and

H2 = {0,2,4,6,8}, ispmorphic to Z5.

Then Z10/H2 is isomorphic to Z2 and the coset 1 + H2 corresponds to 1 in Z2. This explains the third picture.

Also, Z10/H1 is isomorphic to Z5 and the union of cosets 1 + H1, 2 + H1, 3 + H1 and 4 + H1 corresponds to {1,2,3,4} in Z5. This explains the fourth picture.

Return to Other Mod Exercises.