Block Fractals

Exercises - Four Block Configuration Perimeter

From the dimension computation, we expect the perimeter of the limiting shape to be infinite.

Consequently, it suffices to find a collection of edges, each contained in the perimeter of the corresponding level of the four block construction, that diverges to infinity.

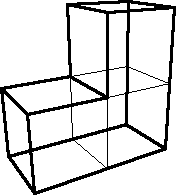
We comapre the perimeter of the four block construction (middle) with that of the three block construction (left).

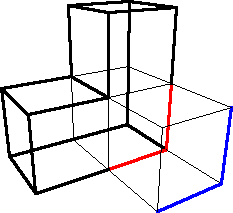
In red are the edges of the three block construction that do not belong to the perimeter of the four block construction.

Note for each red edge there is a corresponding blue edge belonging to the perimeter of the four block construction.

So because the limiting shape of the three block construction has infinite perimeter, the limiting shape of the four block construction must have infinite perimeter.

Another appraoch to see the limiting shape of the four block construction has infinite perimeter is

(1) to note the limiting shape contains a gasket (several in fact: each face is a gasket), and

(2) to recall that the gasket has infinite perimeter.

Return to exercises.